Select a year to see courses
Make the most of NESA Maths Reference Sheet and test your maths skills with these sample questions and explanations for functions, relations and trigonometry!
Join 75,893 students who already have a head start.
"*" indicates required fields
The NESA Maths Reference Sheet is a great resource… if you know how to use it! Navigate functions (including trigonometric ones!) and relations with our Ultimate NESA Maths Reference Sheet Guide.
While memorisation has its place in learning, Matrix recommends that students learn to derive their responses and learn how to apply these formulae correctly. As a bonus, we’ve included a nifty HSC Maths Cheatsheet for you to download and print out!
Download your FREE Maths Formula Handbook
Click on the following formulas to see what they mean and apply them to a practice question!
| Functions |
| \(x=\frac{-b± \sqrt{b^2-4ac}}{2a}\) \(\text{For} \ ax^3 + bx^2 +cx + d = 0 ;\) |
| Logarithmic and Exponential Functions |
| \(\text{log}_a a^x = x = a^{\text{log}_a x}\) |
| Use | Formula | Variables |
| Solve quadratic equations | \(x=\frac{-b± \sqrt{b^2-4ac}}{2a}\) | a, b and c are the coefficients of a quadratic equation in the form:\(ax^2+bx+c=0\) |
Back to top
Next section: Relations
Example 12:
Consider the following equation: \(-2y^2+3y+1=0\)
Find all the solutions of \(y\).
Solution 12:
| Even though the above equation uses \(y\) as a variable instead of \(x\), the quadratic formula works the same way. \begin{align*} Hence, substitute \(a=-2\), \(b=3\) and \(c=1\) into \(y=\frac{-b± \sqrt{b^2-4ac}}{2a}\): \begin{align*} |
Example 13:
\(x=1\) and \(x=3\) are two solutions to the monic equation: \(ax^3 + bx^2 +cx + d = 0\)
Given that the product of the roots is -6, find the coefficients \(a,b,c\) of this cubic polynomial.
Solution 13:
| The question states that the equation is monic. This means that, a=1. \begin{align*} It’s given that \(x=1\) and \(x=3\) are solutions to the cubic equation, \(x=1\) and \(x=3\) are two roots of the equation. Hence, substitute \(α=1\) and \(β=3\) into \(αβγ = -6\): \begin{align*} To find the coefficients b and c (we’ve already found \(a=1\) and \(d=6\)): Substitute \(α=1\), \(β=3\) and \(γ=-2\) into equation 1: \(α+β+γ = -b\) and equation 2: \(αβ+αγ+βγ = c\): \begin{align*} Therefore, the coefficients of the equation \(ax^3 + bx^2 +cx + d = 0\) are \(a=1\), \(b=-2\),\(c=7\) and \(d=6\). |
| Use | Formula | Explanation |
| Cartesian equation of a circle | \((x-h)^2+(y-k)^2=r^2\) | Cartesian equation of a circle centred at (h, k) with radius = r
|
Back to top
Next section: Logarithmic and Exponential Functions
Example 14:
The centre of a circle lies at the intersection of two lines: \(y = x\) and \(y = 2x – 4\)
Given that the area of this circle is \(16π \) units2, find the Cartesian equation of this circle.
Solution 14:
| To find the centre of the circle, first we simultaneously solve the equations \(y = x\) and \(y = 2x – 4\) to find their point of intersection.
\begin{align*} To find the radius r of the circle, we can use the information that the area of this circle is \(16π \) units2. The formula for the area of a circle is: \( A = \pi r^2 \) \begin{align*} The equation of the circle is: \((x-h)^2+(y-k)^2=r^2\) where \((h, k)\) = \((4, 4)\) and \(r = 4\) \begin{align*} |
Example 15:
Given the below right-angle triangle, find the value of the following trigonometric expressions:
a) sinA
b) cos(90°-A)
c) tan90°
Solution 15:
| a) sinA = \(\frac{4}{5}\) b) cos(90°-A) = sinA = \(\frac{4}{5}\) Alternatively, recognise that (90°-A) is an angle in the triangle.
Hence, cos(90°-A) = \(\frac{\text{adj}}{\text{hyp}}\) = \(\frac{4}{5}\) c) tan90° = undefined As we can see in this right angle triangle, the length opposite 90° is the hypotenuse. Hence, the expression \( \text{tan}90° = \frac{\text{opp}}{\text{adj}}\) is undefined. |
Example 16:
Given that the following triangle has an area of \(4 \sqrt{3}\) cm2, find the length x.
Solution 16:
| Substitute \(A= 4 \sqrt{3}\), \(a=3\), \(b=x\) and \(C=60°\) into \(A= \frac{1}{2}ab\text{sin}C\): \begin{align*} |
Example 17:
Find length x. Giver your answer to the nearest 3 decimal places.
The diagram is not to-scale.
Solution 17:
| In order to use the sine rule to find x, we need to know the angle θ that is opposite length x.
\begin{align*} Thus, we can use the sine rule: Substitute \(a=4\), \(A=30°\), \(b=x\), \(B=θ=105°\) into \(\frac{a}{\text{sin}A} = \frac{b}{\text{sin}B}\): \begin{align*} |
Example 18:
a) Sal is fencing a paddock for his sheep. He wants to make the paddock the shape of a sector with a radius of 6 metres. If he has \(12 + 2π\) metres of fencing, what is the angle of the largest sector he can fully enclose? Give your answer as an exact value in radians.
b) Hence, find the maximum area of Sal’s paddock.
Solution 18 a):
The following diagram illustrates the paddock.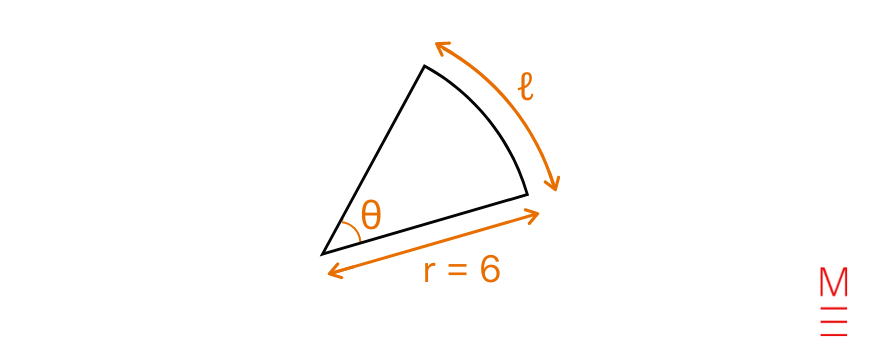 The perimeter of the sector: \(P = r+r+l.\text{ }\)Since Sal is limited to \((12 + 2π)\) metres of fencing, substitute \(P =12 + 2π\) and \(r=6\) (given).\begin{align*}12 + 2π &= 6 + 6 + l \\ l &= 2π \\ \end{align*}Hence, the arc length of the largest sector Sal can enclose is \(2π\). However, this is not our final answer as we are required to find the angle of the largest sector. Substitute \(l = 2π\) and \(r=6\) into \(l=r \theta\):\begin{align*} 2π &= 6 \times \theta \\ \theta &= \frac{π}{3} \\ ∴ \text{Angle of the largest sector} &= \frac{π}{3}^c \\ \end{align*} |
Solution 18 b):
| We are given that the radius of the sector is 6 metres and in part a), we found that the largest possible angle of the sector is \(frac{π}{3}^c\). So, to find the largest area of the sector, substitute \(r=6\) and \(\theta=frac{π}{3}^c\) into \(A= \frac{1}{2} r^2 \theta\): \begin{align*} |
Example 19:
Prove that: \(\text{tan}θ\text{sin}θ + \text{cos}θ = \text{sec}θ\)
Solution 19:
| \begin{align*} LHS &= \text{tan} \theta \text{sin} \theta + \text{cos} \theta \\ \text{Since } \text{tan} \theta = \frac{ \text{sin} \theta }{ \text{cos} \theta }, LHS &= \frac{ \text{sin} \theta }{ \text{cos} \theta } \times \text{sin} \theta + \text{cos} \theta \\ &= \frac{ \text{sin}^2 \theta }{ \text{cos} \theta } + \frac{ \text{cos}^2 \theta }{ \text{cos} \theta } \\ &= \frac{ \text{sin}^2 \theta + \text{cos}^2 \theta }{ \text{cos} \theta } \\ \text{Since } \text{sin}^2 \theta + \text{cos}^2 \theta=1, &= \frac{1}{ \text{cos} \theta } \\ &= \text{sec} \theta \\ &= RHS \\ ∴ \text{tan} \theta \text{sin} \theta + \text{cos} \theta = \text{sec} \theta \\ \end{align*} |
Expanding a sum of angles in a trigonometry function
Example 20:
Find the exact value of \(cos75º\).
Solution 20:
| \begin{align*} \text{cos}75º &= \text{cos}(45+30)º \\ \text{Since } \text{cos}(A+B)=\text{cos}A\text{cos}B-\text{sin}A\text{sin}B \\ \text{cos}(45+30)º &= \text{cos}45\text{cos}30-\text{sin}45\text{sin}30 \\ &= \frac{ \sqrt{2}}{2} \times \frac{ \sqrt{3}}{2} – \frac{ \sqrt{2}}{2} \times \frac{1}{2} \\ &= \frac{ \sqrt{6}}{4} – \frac{ \sqrt{2}}{4} \\ ∴ \text{cos}75º &= \frac{ \sqrt{6} – \sqrt{2}}{2} \\ \end{align*} |
T-formula
Example 21:
Given \(2\text{cos}2B – \text{sin}2B = 1\), solve for \(B\), \(0 \le B \le 180°\):
If necessary, give your answers to the nearest 2 decimal places.
Solution 21:
| \begin{align*} \text{Let } t &= \text{tan}B \\ ⇒ \text{sin}2B &= \frac{2t}{1+t^2}\\ \text{cos}2B &= \frac{1-t^2}{1+t^2}\\ \text{tan}2B &= \frac{2t}{1-t^2}\\ \\ \text{Given that }2\text{cos}2B – \text{sinB}2B &= 1 \\ ⇒ 2 \times \frac{1-t^2}{1+t^2} – \frac{2t}{1+t^2} &= 1 \\ (1+t^2) \times (2 \times \frac{1-t^2}{1+t^2} – \frac{2t}{1+t^2}) &= (1+t^2) \times 1 \\ 2 (1-t^2) – 2t &= 1+t^2 \\ 2 – 2t^2 – 2t &= 1+t^2 \\ -3t^2 – 2t + 1 &= 0 \\ 3t^2 + 2t – 1 &= 0 \\ (3t-1)(t+1) &= 0 \\ 3t-1 &= 0 \text{ or } t+1=0 \\ t &= \frac{1}{3} \text{ or } t=-1 \\ ⇒ \text{tan}B &= \frac{1}{3} \text{ or } \text{tan}B=-1 \\ ∴ B &= \text{tan}^{-1} \frac{1}{3} \text{ or } B = 180 + {tan}^{-1}(-1) \text{ (since 0<B<180°)} \\ ∴ B &= 18.43° \text{ or } 135° \\ \end{align*} |
Manipulating a product of trigonometric functions into a sum of trigonometric functions
Example 22:
Find the exact value of the product of \(\text{cos}15º\) and \(\text{sin}45º\).
Solution 22:
| \begin{align*} \text{Since } \text{cos}A\text{sin}B & =\frac{1}{2}[\text{sin}(A+B)-\text{sin}(A-B)] \\ ⇒ \text{cos}15º\text{sin}45º &= \frac{1}{2}[\text{sin}(15+45)-\text{sin}(15-45)] \\ &= \frac{1}{2}[\text{sin}(60)-\text{sin}(-30)] \\ &= \frac{1}{2}[\frac{ \sqrt{3}}{2}+ \frac{1}{2} ] \\ ∴\text{cos}15º\text{sin}45º &= \frac{\sqrt{3}+1}{4} \end{align*} |
Double angle identities
| Identity | Variables |
| \(\text{sin}^2nx=\frac{1}{2}(1-\text{cos}2nx)\) | \(x, \ n ∈ R\) |
| \(\text{cos}^2nx=\frac{1}{2}(1+\text{cos}2nx)\) |
Example 23:
Evaluate \(2(\text{sin}22.5º)^2\). Give your answer in exact form.
Solution 23:
| \begin{align*} \text{Since } \text{sin}^2nx &=\frac{1}{2}(1-\text{cos}2nx) \\ ⇒ 2(\text{sin}22.5º)^2 &= 2 \times \frac{1}{2}(1-\text{cos}45º) \\ &= 1- \frac{ \sqrt{2}}{2} \\ &= \frac{ 2- \sqrt{2}}{2}\\ ∴2(\text{sin}22.5º)^2 &= \frac{ 2- \sqrt{2}}{2} \\ \end{align*} |
Written by Matrix Maths Team
The Matrix Maths Team are tutors and teachers with a passion for Mathematics and a dedication to seeing Matrix Students achieving their academic goals.
© Matrix Education and www.matrix.edu.au, 2023. Unauthorised use and/or duplication of this material without express and written permission from this site’s author and/or owner is strictly prohibited. Excerpts and links may be used, provided that full and clear credit is given to Matrix Education and www.matrix.edu.au with appropriate and specific direction to the original content.
