Welcome to Matrix Education
To ensure we are showing you the most relevant content, please select your location below.
Select a year to see courses
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Learn online or on-campus during the term or school holidays
Get HSC Trial exam ready in just a week
Get HSC exam ready in just a week
Select a year to see available courses
Science guides to help you get ahead
Science guides to help you get ahead

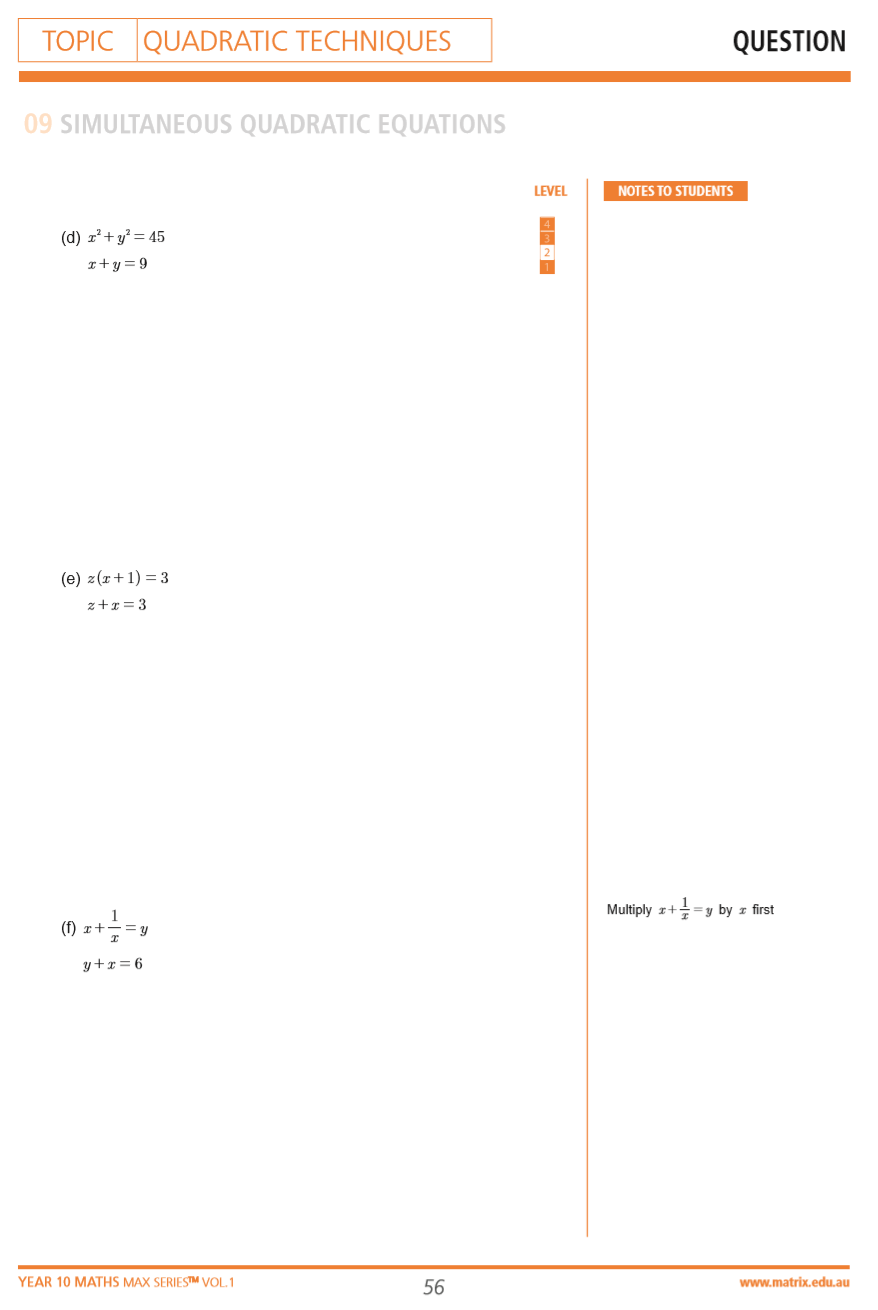
In this article, we’ll build on what you learned last year when you studied simultaneous equations. Now we’ll explain quadratic equations and non-linear simultaneous equations.
We will step you through the processes for solving different quadratic and non-linear simultaneous equations. Then you can test your skills and knowledge on our concept check questions. This guide provides you with worked examples so you can see where you went wrong, or right!
We will teach you how to solve non-linear simultaneous equations and quadratic equations.
This page will also teach you how to interpret a graph to inspect how many solutions there are to an equation without solving the equation.
Test your skills in solving quadratic equations and graphs. Fill out your details below to get this resource emailed to you. "*" indicates required fields
Free Year 10 Quadratic Equations and Graphs Worksheet

Free Year 10 Quadratic Equations and Graphs Worksheet
This article will cover the following syllabus outcomes:
Stage 5.3: Solves complex linear, quadratic, simple cubic and simultaneous equations and rearranges literal equations. (MAS.3-7NA)
Solve simultaneous equations, where one equation is non-linear, using algebraic and graphical techniques, including the use of digital technologies
These Outcomes are building on the previous unit in Year 9. Here you will learn to solve equations algebraically and graphically with an \(x^2\) term. For example, \(y=3x+2\) and \(x^2+y^2=1\).
Students should be familiar with basic algebraic techniques and simple arithmetic.
Knowledge of basic curve sketching will also be required.
For harder problems, students should also know how to sketch more complicated functions such as hyperbolae, circles, semi-circles, exponential functions and cubic curves – but students will still understand the theory behind the content without knowing how to sketch these harder functions as they may instead use technology to do so.
We have learnt the graphical, substitution and elimination method for linear sets of equations. Now we can apply the same techniques to solve non-linear equations.
From sketching a graph, we can see that there are two points of intersection;
But it is not at all obvious what the exact points of intersection are. (Solving this problem does not need a graph).
Elimination method is not so useful here since one equation involves \(x\) and \(y\) while the other involves \(x^2\) and \(y^2\).
So we will use the substitution method.
\(Substituting y from (1) into (2), we obtain
\(Substitute these \(x\) values back into either (1) or (2) (we immediately see that (1) is easier) to solve for the corresponding value for \(y\).
When \(x=-\frac{1}{2}-\frac{\sqrt{7}}{2} ,y=\frac{1}{2}-\frac{\sqrt{7}}{2}\) and when \(x=\frac{\sqrt{7}}{2}-\frac{1}{2},y=\frac{1}{2}+\frac{\sqrt{7}}{2}\)
and thus \((-\frac{1}{2}- \frac{\sqrt7}{2}, \frac{1}{2}-\frac{\sqrt7}{2})\) and \((\frac{{\sqrt7}}{2}-\frac{1}{2},\frac{1}{2}+\frac{\sqrt7}{2})\) are the points of intersection.
It was said earlier that “the solution to a set of simultaneous equations is the common point of intersection”.
This observation makes it very easy to find the number of solutions to an equation without having to solve it.
The number of solutions to the equation
\(ax+b=cx+d\)or the pair of equations:
\(y=ax+b\) and \(y=cx+d\)
is the number of points of intersection caused by graphing \(y=ax+b\) and \(y=cx+d\) on the same number plane.
In general, for students who have seen functional notation, the number of solutions to an equation
\(f(x)=g(x)\)is simply the number of points of intersection caused by graphing \(y=f(x)\) and \(y=g(x)\) on the same number plane.
How many solutions are there to the set of simultaneous equations:
\(2x+y-20=0\) and \(6x+3y=1\)
Since both lines have the same gradient, they are parallel.
Parallel lines never intersect so there will be no solutions.
Graphing the two lines, we can see this visually
Using technology, how many solutions are there to the simultaneous equations
\(y^2=4x\) and \( x^3+y^3=100\)
The graphing package Desmos gives the following graph:
Counting the points of intersection, we can see that there are 2 solutions.
1. Solve for the points of intersection that the line \(y=2x+1\) makes with the hyperbola \(y=\frac{1}{x}\)
2. Solve for the points of intersection that the line \(y=x-1\) makes with the circle \(x^2+y^2=1\)
3. By sketching the two curves, explain why \(x^2=x-1\) has no solutions
4. By sketching the two lines, explain why the pair of equations \(x+2y=1\) and \(2x+4y=5\) have no solution when solved simultaneously.
5. By using technology or your knowledge of curve sketching, sketch \(y=\frac{1}{2} x^2\) and \(x^2+y^2=1\) on the same number plane and hence state the number of solutions for the equation \(x^2+\frac{1}{4} x^4=1\)
6. It is actually possible to solve more than two equations simultaneously.
The general equation of a parabola is \(y=ax^2+bx+c\).
Given that a particular parabola passes through the points \((1,2), (-1,0)\) and \((2,9)\), show that this results in the three equations:
\(
a+b+c=2…(1) \\
a-b+c=0…(2) \\
4a+2b+c=9…(3) \\
\)
7. CHALLENGE
Use a clever elimination by considering (a) and (b) to solve for \(b\) immediately in Question 9 above. Then solve for \(a\) and \(c\) in order to give the equation of the parabola in Question 9.
1.
\(
y= \frac{1}{x}…(1) \\
y=2x+1…(2) \\
\)
Substitute (1) into (2):
\(
\frac{1}{x}=2x+1 \\
2x^2+x-1=0 \\
(x+1)(2x-1)=0 \\
x=-1, \frac{1}{2} \\
\)
When \(x=-1, y=-1\) and when \(x= \frac{1}{2}, y=2\)
Thus \((-1,-1)\) and \((\frac{1}{2},2)\) are the points of intersection.
2.
\(
y=x-1…(1) \\
x^2+y^2=1 …(2) \\
\)
Substitute for \(y\) from (1) into (2):
\(
x^2+(x-1)^2=1 \\
2x^2-2x+1=1 \\
x(x-1)=0 \\
x=0,1 \\
\)
When \(x=0,y=-1\) and when \(x=1,y=0\)
Thus \((0,-1)\) and \((1,0)\) are the points of intersection
3.
We first sketch \(y=x^2\) and \(y=x-1\) on the same number plane:
There are no points of intersection, thus there are no solutions.
4.
By sketching the two lines, explain why the pair of equations \(x+2y=1\) and \(2x+4y=5\) have no solution when solved simultaneously.
The two lines are parallel and thus will never intersect. So the equations will have no solution when solved simultaneously.
5.
We see that there are two solutions to the pair of simultaneous equations. Substituting the parabola into the circle, we get that
\(
x^2+(\frac{1}{2} x^2 )^2=1 \\
x^2+\frac{1}{4} x^4=1 \\
\)
And so this equation will have two solutions.
6.
Since the parabola passes through the three points, we should be able to substitute each point into the general equation of the parabola to obtain the three equations.
\((1,2)⇒2=a×1^2+b×1+c \\
(-1,0)⇒0=a×(-1)^2+b×(-1)+c \\
(2,9)⇒9=a×2^2+b×2+c \\
\)
which simplifies to the three equations needed.
7.
\(
a+b+c=2…(1) \\
a-b+c=0…(2) \\
4a+2b+c=9…(3) \\
\\
(1)-(2): 2b=2⇒b=1\\
\)
Now we can rewrite (1),(2) and (3)
\(
a+c=1 …(1) \\
a+c=1 …(2) \\
4a+c=7 …(3) \\
\)
(1) and (2) give us the same information, so we now need to use (3) as well.
(3)-(1) gives
\(3a=6⇒a=2\)So (1) can now be solved for c:
\(2+c=1⇒c=-1\)Hence \(a=2,b=1,c=-1\)
Thus the parabola that passes through the points \((1,2),(-1,0)\) and \((2,9)\) is
\(y=2x^2+x-1\)

In our Year 10 Maths Course, we will teach you key Maths concepts and provide you with questions to practice. Learn more now!
Gain the confidence to ace your next Maths assessment!
Develop your skills. Boost your confidence. Raise your rank with our Maths On Campus Term Course.
In our next article, we discuss probability. Read on if you want to increase your odds of acing Year 10 Maths!
© Matrix Education and www.matrix.edu.au, 2025. Unauthorised use and/or duplication of this material without express and written permission from this site’s author and/or owner is strictly prohibited. Excerpts and links may be used, provided that full and clear credit is given to Matrix Education and www.matrix.edu.au with appropriate and specific direction to the original content.